
We thus avoid the unstable case of taking the cross product of two nearly-aligned vectors. Dot product of two perpendicular vectors: Calculate the perpendicular vectors. In this least-orthogonal case, we're still quite orthogonal given that our unit vector has all but one dimension 0 whereas vec has all equal. It's thus guaranteed to be loosely orthogonal to vec before taking the cross product, with least orthogonality in the case where all dimensions of vec are equal. 1 Then take the cross product of the arbitrary vector with the input vector. For instance, pick (1, 0, 0) unless the vector is (x, 0, 0) for some x otherwise pick (0, 1, 0). For 'something rigid', you can have a fixed rule. Consider that our unit vector is large in only a single dimension and that that dimension corresponds to the dimension where vec was small. Pick an arbitrary vector that is not collinear with the given vector. Consider now that the cross product is numerically unstable only when the two vectors are very closely aligned. The cross product of this with vec is orthogonal to vec by defn of cross product.
The orientation of the resulting normal vector points to the left from. Consider the vector w w extending from the quarterback’s arm to a point directly above the receiver’s head at an angle of 30 30 (see the following figure). We then scale the vector appropriately so that it has the right magnitude. So, could you help me to find the vector which is perpendicular to another given vector PLZ Anyone helps me. However, my final goal is to find the intersection point of above two lines. Suppose that v2 is passing through the second point x2,y2,z2. We then have a unit vector with a single non-zero dimension that corresponds to a dimension of minimum magnitude in vec. Determines the 2D unit normal vector to line p1,p2. The first thing we want to do is find a vector in the same direction as the velocity vector of the ball. Now I need a second vector, v2 which is perpendicular to the v1. Given a vector v in the space, there are infinitely many perpendicular vectors. A vector can be represented in both two dimensional and three-dimensional. A normal vector is a perpendicular vector. Return cross(vec, Vec3D(int(b0), int(b1), int(b2))) Įxactly 1 and only 1 of the bools get set bN gets set if dimension N has magnitude strictly less than all subsequent dimensions and not greater than all previous dimensions. The magnitude of each vector is given by the formula for the distance between. Vec3D arbitrary_orthogonal(Vec3D vec)īool b0 = (vec < vec) & (vec < vec) īool b1 = (vec <= vec) & (vec < vec) īool b2 = (vec <= vec) & (vec <= vec)

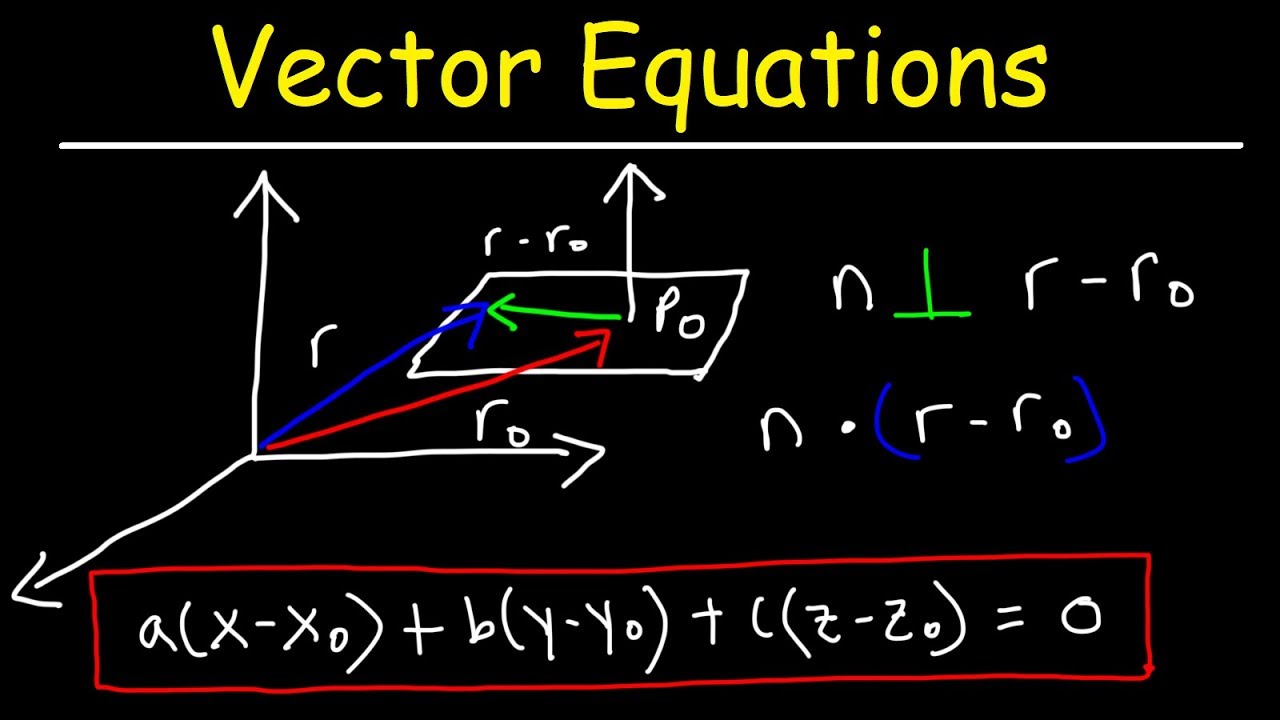
Assume that Vec3D is a three dimensional vector of arbitrary numerical type. The result is the vector orthogonal to the plane.I believe that this should produce an arbitrary vector that is perpendicular to the given vector vec while remaining numerically stable regardless of the angle of vec (assuming that the magnitude of vec is not close to zero). If we only have the three points, then we need to use them to find the two vectors that lie in the plane, which we’ll do using these formulas: Compute the outer product of two vectors. Other times, we’ll only be given three points in the plane. The term matrix as it is used on this page indicates a 2d numpy.array object, and not a numpy.matrix object.


 0 kommentar(er)
0 kommentar(er)
